Post History
This is a fixed output challenge. Output a textual representation of a chessboard hosting 8 queens, none of which are attacking each other. There are 92 ways of arranging them, 12 if rotations and...
#1: Initial revision
by
trichoplax
·
2022-09-29T12:13:45Z (over 1 year ago)
8 coexisting queens
This is a fixed output challenge. Output a textual representation of a chessboard hosting 8 queens, none of which are attacking each other. There are 92 ways of arranging them, 12 if rotations and reflections are discounted. You may choose any 1 of these arrangements. You are not required to calculate or search for a valid arrangement. Hardcoding your choice of arrangement is one possible valid solution. ## Input - There is no input for this challenge ## Output - 8 newline separated lines of 8 characters, with an optional trailing newline - Each character is either a queen or an empty square. You may choose any two distinct characters to represent a queen and an empty square - No more than 1 queen appears in each row, column, and diagonal - There are 8 queens in total - Your output must be the same each time (your code must have deterministic output, even if it uses a probabilistic approach internally) ## Examples The examples show a queen as `Q` and an empty square as `#`. There are 12 examples, one for each of the fundamental solutions (before rotation and reflection). Any combination of rotating and reflecting one of these examples is also a valid solution. ```text ###Q#### ######Q# ##Q##### #######Q #Q###### ####Q### Q####### #####Q## ####Q### #Q###### ###Q#### ######Q# ##Q##### #######Q #####Q## Q####### ###Q#### #Q###### ######Q# ##Q##### #####Q## #######Q ####Q### Q####### ###Q#### #####Q## #######Q ##Q##### Q####### ######Q# ####Q### #Q###### ##Q##### #####Q## #######Q Q####### ###Q#### ######Q# ####Q### #Q###### ####Q### ##Q##### #######Q ###Q#### ######Q# Q####### #####Q## #Q###### ####Q### ######Q# ###Q#### Q####### ##Q##### #######Q #####Q## #Q###### ###Q#### Q####### ####Q### #######Q #####Q## ##Q##### ######Q# #Q###### ##Q##### #####Q## ###Q#### Q####### #######Q ####Q### ######Q# #Q###### #####Q## #Q###### ######Q# Q####### ###Q#### #######Q ####Q### ##Q##### ###Q#### ######Q# Q####### #######Q ####Q### #Q###### #####Q## ##Q##### #####Q## ###Q#### ######Q# Q####### #######Q #Q###### ####Q### ##Q##### ``` The 12 fundamental solutions were taken from the [Wikipedia page for the 8 queens puzzle](https://en.wikipedia.org/wiki/Eight_queens_puzzle#Constructing_and_counting_solutions_when_n_=_8) which also provides them as images: [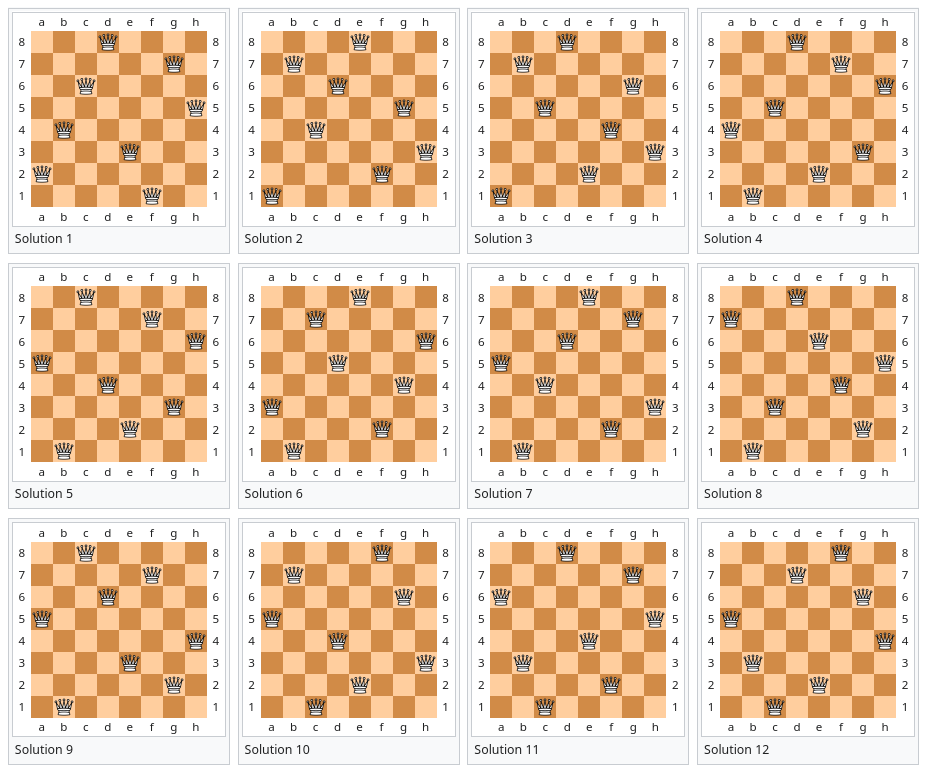](https://en.wikipedia.org/wiki/Eight_queens_puzzle#Constructing_and_counting_solutions_when_n_=_8 "View the images on Wikipedia") ## Scoring Despite there being 92 valid outputs, this is a standard code golf challenge. Your score is the number of bytes in your code. > Explanations in answers are optional, but I'm more likely to upvote answers that have one.